Solutions for the Right Wiggly Triangles

Part 1

Suppose there are

t right wiggly triangles in the circle
p points inside the circle but not on the outside where the triangles meet
q points on the circle itself (and therefore also q lines)
e edges inside the circle - not counting the q on the outside

Then we have:

Number of faces = t+1 including the outside
Number of edges = e+q
Number of vertices = p+q

Firstly add up the angles of the triangles. It is 180° per triangle. Also it is 360° per interior point and 180° for each on the circle.

180t = 360p + 180q

or simplifying

t = 2p + q

Now count the total number of sides of the triangles. Each interior side is on two triangles whereas those on the circle are only on one triangle.

3t = 2e + q

Subtracting the previous equation t=2p+q from this gives;

2t = 2e - 2p

Which is the same as

t + p = e

However by Euler's formula F+E=V+2 therefore

t+1 + p+q = e+q + 2

which gives

t + p = e + 1

and subtracting t+p=e from this gives the classical contradiction

0 = 1

QED we can't divide up a circle into right wiggly triangles, it is just plain impossible.

Part 2

You'll like this (not a lot!).

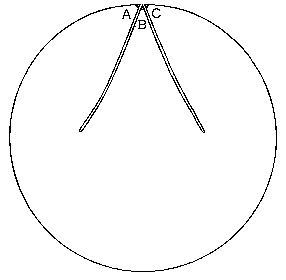
A single right wiggly triangle is sufficient. The sides AB and BC are bent back on themselves so corners A, B and C all meet at a point. AC forms the outside of the circle. Angles A+B+C make up 180°.

problem case, single right wiggly triangle making a circle